How To Find X In A Circle

Let's work through four different examples of how to detect the equation of a circle

Example

What is the equation of the circle?

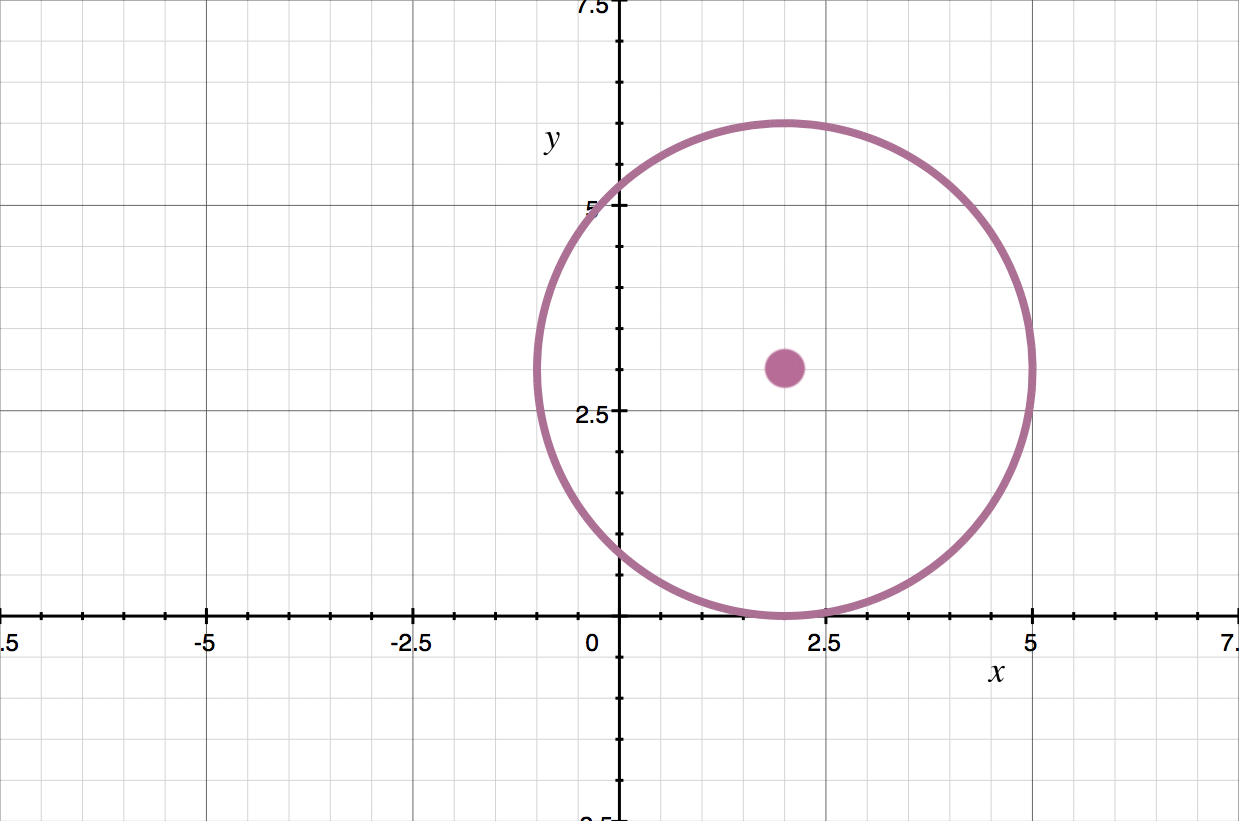
equation of the circle in the coordinate plane

We need to find the equation of a circle in the form ???(x-h)^2+(y-m)^2=r^2???, which ways we need to find the center bespeak and the length of the radius.

The center is at ???(2,three)???, so ???h=2??? and ???k=3???.

Now let's count from the center to a point on the circumference to discover the length of the radius.

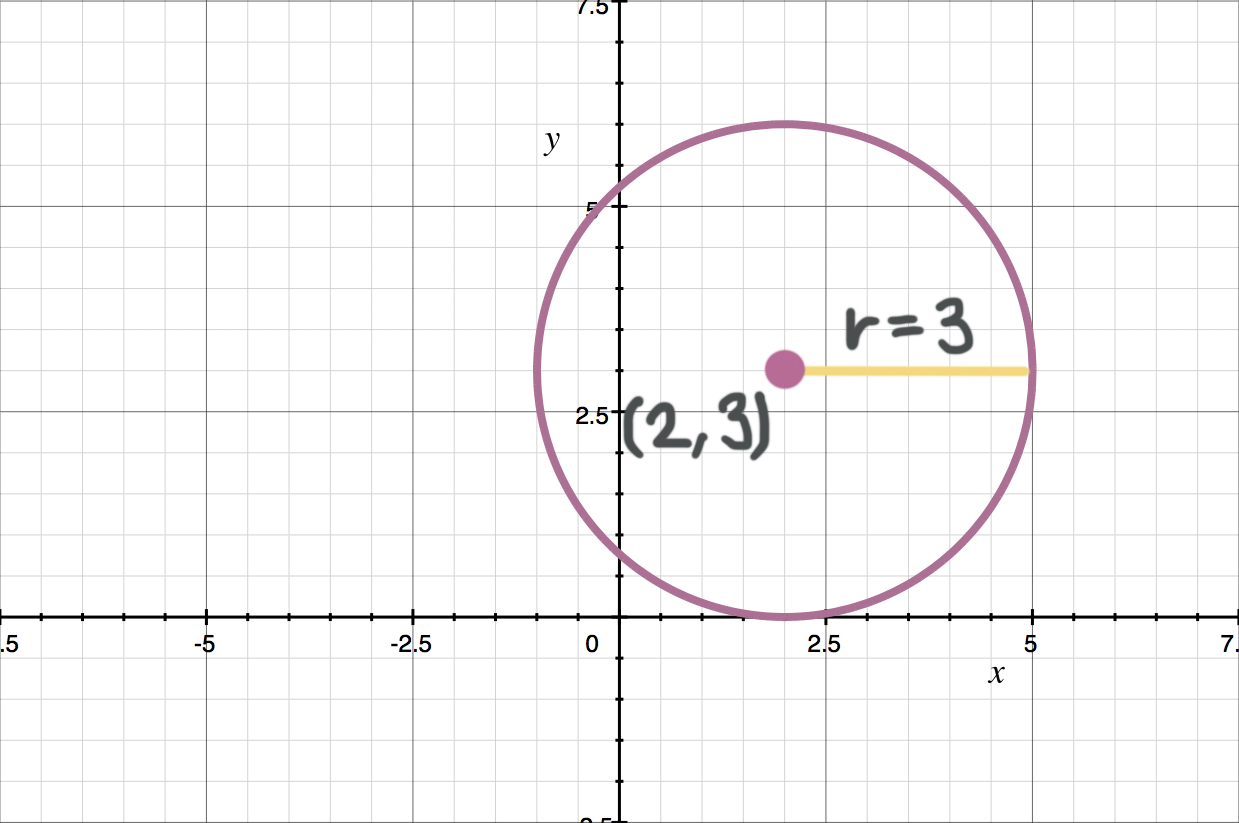
The radius is ???3??? then ???r=3???. Now let's plug everything into the standard form of a circumvolve.

???(10-two)^2+(y-3)^ii=iii^2???

???(x-2)^ii+(y-3)^2=9???

Sometimes nosotros want to know the center and radius of a circle given the equation of the circle.

Example

What is the eye and radius of the circle?

???{{10}^{two}}+{{(y-3)}^{two}}=27???

We can rewrite the equation as

???{{(10-0)}^{ii}}+{{(y-three)}^{2}}=27???

Which lets u.s.a. identify ???h=0??? and ???k=iii???, and then the center is at ???(0,iii)???. And the radius is and so ???\sqrt{27}???, and then

???r=\sqrt{27}???

???r=\sqrt{9\cdot three}???

???r=\sqrt{9}\cdot \sqrt{iii}???

???r=3\sqrt{iii}???

Sometimes nosotros want to know the -intercepts of a circle.

Equation of a circle for Geometry.jpg — A circle can be defined by a middle signal and a radius of a certain length.

Example

What are the ???x???-intercepts of the circle?

???{{(x-2)}^{ii}}+{{(y+i)}^{two}}=16???

At the ???x???-intercepts, ???y=0???, and so let ???y??? be ???0???, and rewrite the equation.

???{{(x-2)}^{2}}+{{(y+ane)}^{2}}=16???

???{{(10-ii)}^{two}}+{{(0+1)}^{2}}=16???

???{{(x-ii)}^{two}}+1^ii=xvi???

???{{x}^{2}}-4x+4+1=16???

???{{10}^{2}}-4x+v=16???

???{{x}^{2}}-4x-eleven=0???

If you can't factor the equation to solve for ???10??? you can use the quadratic formula. In this instance, ???a=i???, ???b=-4???, and ???c=-11???.

???x=\frac{-b\pm \sqrt{{{b}^{2}}-4ac}}{2a}???

???x=\frac{4\pm \sqrt{{{(-4)}^{2}}-4(1)(-eleven)}}{2(1)}???

???10=\frac{4\pm \sqrt{60}}{ii}=\frac{iv\pm \sqrt{4\cdot fifteen}}{2}=\frac{4\pm two\sqrt{fifteen}}{ii}=2\pm \sqrt{15}???

These are the ???ten???-intercepts.

Sometimes to find out information nearly a circumvolve yous'll demand to know how to complete the foursquare.

Example

Detect the center and radius of the circumvolve.

???x^2+y^2+24x+10y+160=0???

In order to find the center and radius, we need to modify the equation of the circle into standard course, ???(x-h)^2+(y-m)^2=r^2???. In guild to get the equation into standard form, we accept to complete the square with respect to both variables.

Grouping ???x???'due south and ???y???'southward together and moving the constant to the right side, we get

???(10^2+24x)+(y^2+10y)=-160???

Completing the square requires us to take the coefficient on the outset degree terms, divide them by ???2???, so square the effect earlier adding the result back to both sides.

The coefficient on the ???10??? term is ???24???, and so

???\frac{24}{2}=12\quad\rightarrow\quad12^2=144???

The coefficient on the ???y??? term is ???10???, so

???\frac{x}{two}=5\quad\rightarrow\quad5^2=25???

So we add ???144??? inside the parentheses with the ???ten??? terms, ???25??? inside the parenthesis with the ???y??? terms and also add ???144??? and ???25??? to the right side with the ???-160???.

???(10^two+24x +144)+(y^2+10y+25)=-160 + 144+25???

???(10+12)^2+(y+v)^ii=9???

Therefore, the center of the circumvolve is at ???(h,1000)=(-12,-5)??? and its radius is ???r=\sqrt{ix}=three???.